Empirical Demand Functions: Linear and Nonlinear Regression Analyses
- Anna Rizza Bancolita Celoso
- Jun 15, 2014
- 2 min read
Here's a video I included in this report for the learning value it adds in terms of its discussion on the importance and limitations of Demand Forecasting, and the criteria of a good forecasting method.
Before we proceed to Empirical Demand Function, recall the determinants of Quantity Demanded.

LINEAR EMPIRICAL DEMAND FUNCTION OR SPECIFICATION
The simplest demand function is one that specifies a linear relation. In linear form, the Empirical Demand Function is specified as,

where the parameters:
b = change in Q/change in P, assumed to be negative
c = change in Q/change in M > or < 0, if the good is normal or inferior, respectively
d = change in Q/change in P of related goods > or < 0, if related good is a substitute or complement, respectively
e = change in Q/change in N, assumed to be positive
This equation is known as the LINEAR EMPIRICAL DEMAND FUNCTION OR SPECIFICATION. It is a demand function with varying elasticity. When price and quantity observations are SPREAD OVER a wide range of values, ELASTICITY is more likely to VARY.
NOTE: The "hat" (^) symbol is used over the variables representing the parameters to be statistically or empirically estimated in demand elasticity and estimation.
Price, Income and Cross-Price Elasticity
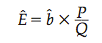
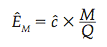
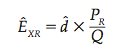
NONLINEAR EMPIRICAL DEMAND FUNCTION OR SPECIFICATION
The most commonly used nonlinear demand function is the LOG-LINEAR (or constant elasticity) FORM, which is specified as,

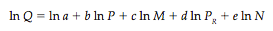
The second function is just the first specification expressed in natural logarithms.
When sample data are clustered over a narrow price (and quantity) range, NONLINEAR EMPIRICAL DEMAND SPECIFICATION is appropriate.
HOW TO ESTIMATE THE PARAMETERS OF THE EMPIRICAL DEMAND FUNCTION?
We are going to use these regression methods for estimating demand function for single price-setting firm.
NOTE: Estimating industry demand requires more advanced statistical methods.
Defining and collecting data to estimate even a simple general demand function requires CAREFUL CONSIDERATION OF NUMEROUS FACTORS, like geographic boundaries of the product market, or whether the market is growing (or shrinking) in size.
The following case example is provided to illustrate how demand specification was chosen to estimate the demand for various imported goods for a time in Trinidad and Tobago (RECALL: the importance of demand forecasting as discussed in the introductory video). It further shows an application of the Log-Linear Demand Model (nonlinear demand function).

























Comments